[10000印刷√] reflection across x axis vs y axis 267880-Reflection across the y axis vs x axis

Just like looking at a mirror image of yourself, but flippeda reflection point is the mirror point on the opposite side of the The reflection of point (x, y) across the yaxis is (x, y) Read full answer Thanks (24) Do you have your own answer or clarification?6) reflection across the xaxis x y B W Z Y 7) reflection across the yaxis x y J R E 8) reflection across the xaxis x y R V C 9) reflection across the yaxis x y L

Stretching And Reflecting Transformations Read Algebra Ck 12 Foundation

Reflection across the y axis vs x axis

Reflection across the y axis vs x axis- That is really a specific example of reflection over a line, where the line happens to be the x axis (ie y = 0x 0) If you want to reflect over another line, you can use a matrix The code below preserves previous transformations on the display object, and reflects it over a line passing through the origin var mNumber = 025; Reflect the shape in the yaxis First go over the x – axis with a different colour so you know exactly where the mirror line is Corner A is 3 squares to the yaxis, so corner A on the reflected shape will be 4 squares away from the yaxis Corner B is 5 squares to the yaxis, so corner B on the reflected shape will be 5 squares away from

Reflections Across The X And Y Axis Geogebra

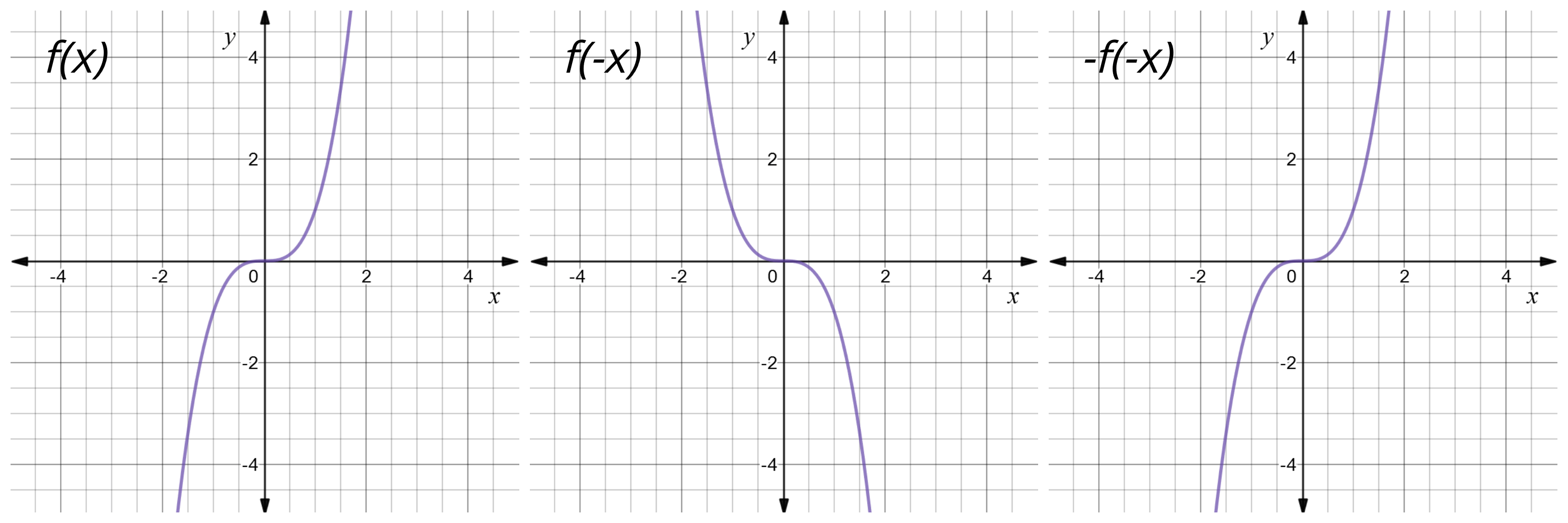
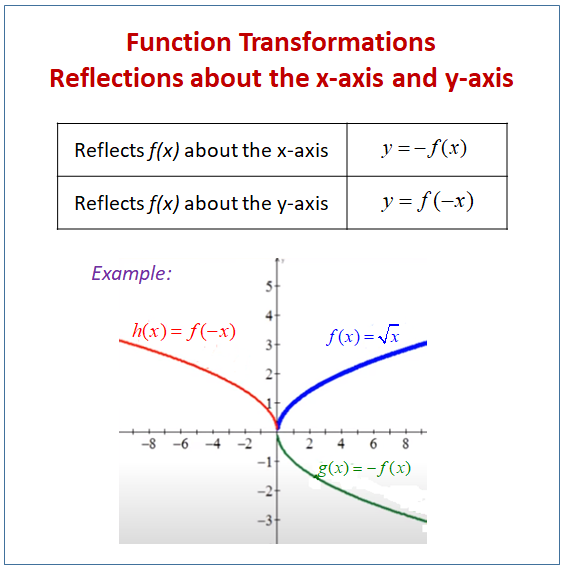
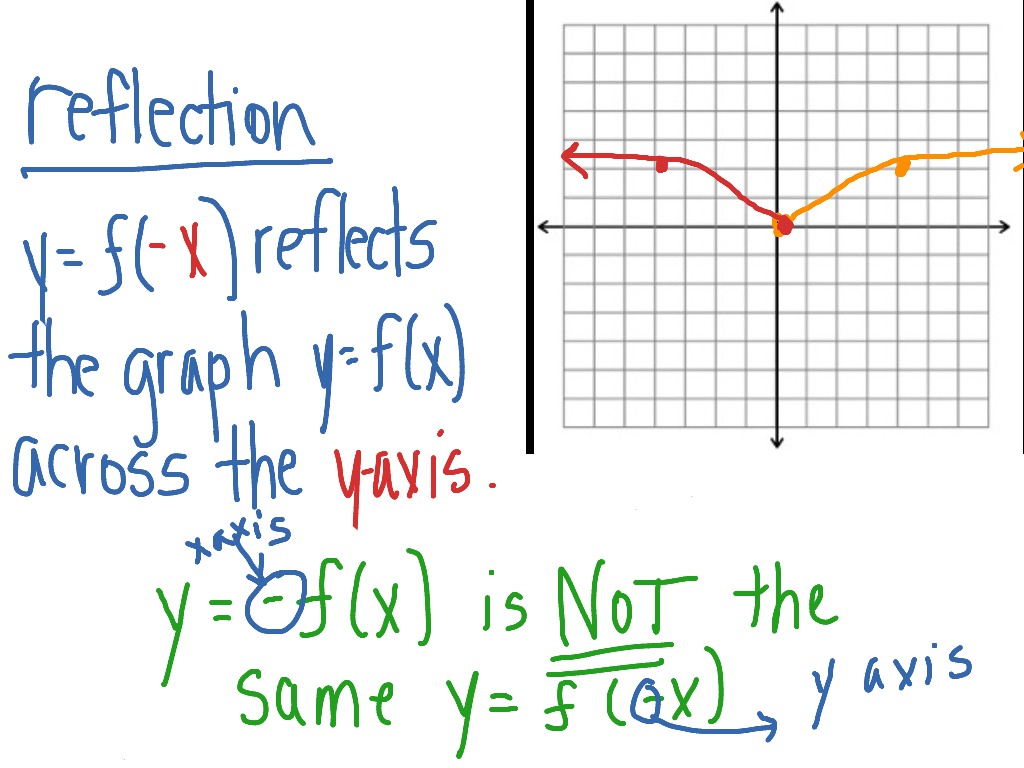
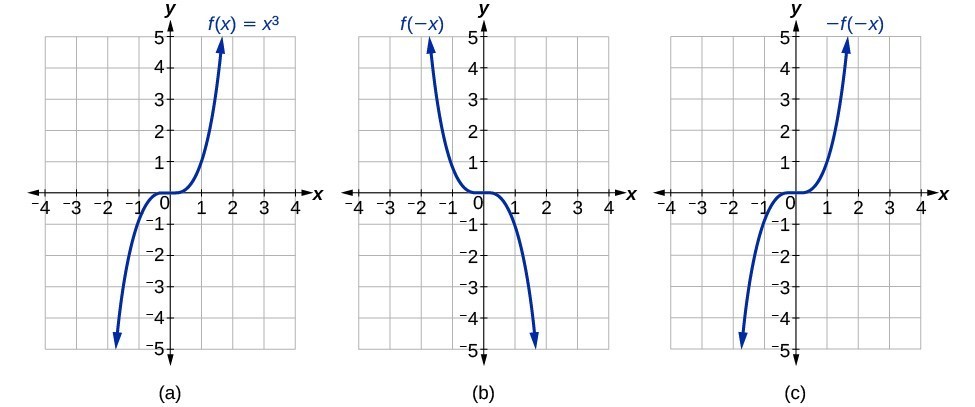
A math reflection flips a graph over the yaxis, and is of the form y = f (x) Other important transformations include vertical shifts, horizontal shifts and horizontal compression Let's talk about reflections Now recall how to reflect the graph y=f of x across the x axis Welcome to The Reflection of 3 Vertices Over the x or y Axis (A) Math Worksheet from the Geometry Worksheets Page at MathDrillscom This math worksheet was created on and has been viewed 149 times this week and 343 times this month It may be printed, downloaded or saved and used in your classroom, home school, or other educationalThe best way to practice drawing reflections across the yaxis is to do an example problem Example Given the graph of y = f (x) y = f(x) y = f (x) as shown, sketch y = − f (x) y = f(x) y = − f (x) Remember, the only step we have to do before plotting the − f (x)f(x) − f (x) reflection is simply divide the ycoordinates of easytodetermine points on our graph above by (1) When

Background Tutorials Use a pair of perpendicular number lines, called axes, to define a coordinate system, with theTo reflect the absolute value function over the xaxis, we simply put a negative sign before the symbol (in this case the absolute value bars) Our new equation would be y = Ix3I Check the graphs in your calculator, they should look like a mirror image of each other, reflected over the xaxis Now try reflecting reciprocal y = 1/x 4Reflections and Rotations Summary Reflections and Rotations Reflections and Rotations We can also reflect the graph of a function over the xaxis (y = 0), the yaxis(x = 0), or the line y = x Making the output negative reflects the graph over the xaxis, or the line y

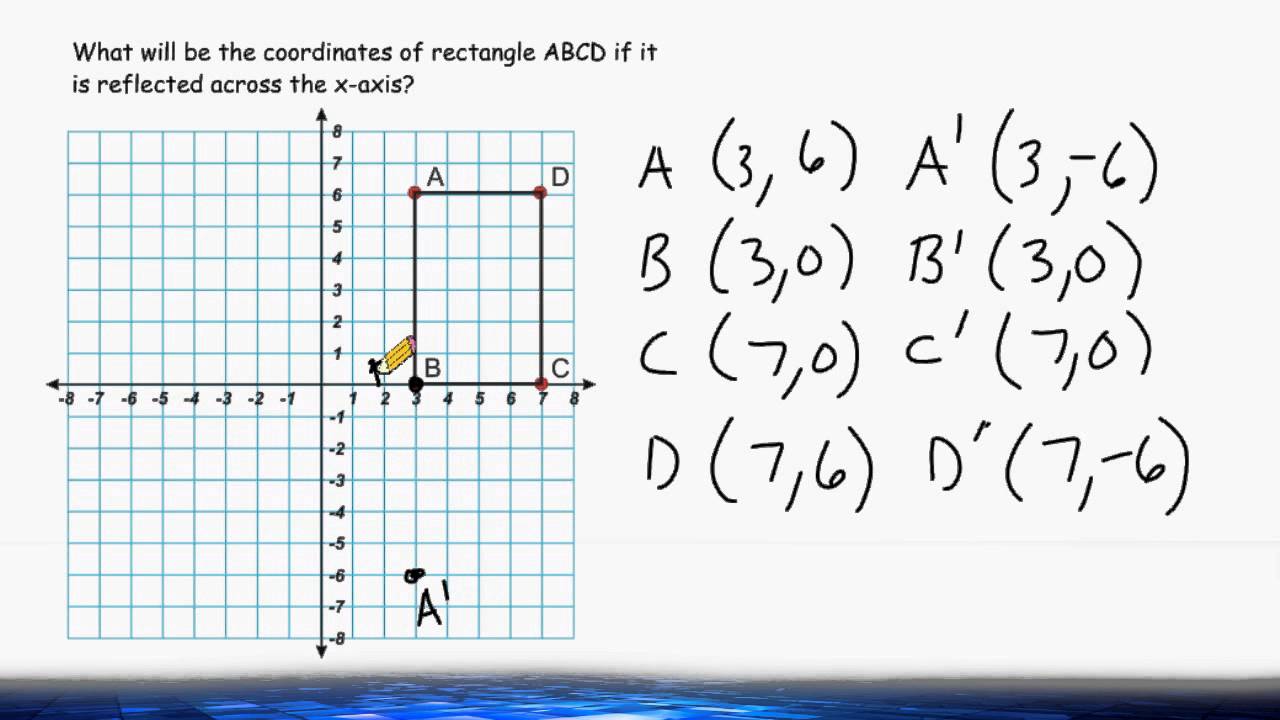
Just about everything in math has a name!Find the vertices of triangle P'Q'R' after a reflection across the xaxis Then graph the triangle and its image Solution Step 1 Apply the rule to find the vertices of the image Since there is a reflection across the xaxis, we have to multiply each ycoordinate by 1 That is, (x, y) > (x, y) Step 2 P(2, 5) > P'(2, 5)First, determine the original coordinate points For this example, we will say the coordinate points are

Reflection Over The X And Y Axis The Complete Guide Mashup Math

Reflecting A Triangle Across The X Axis And The Y Axis Geogebra

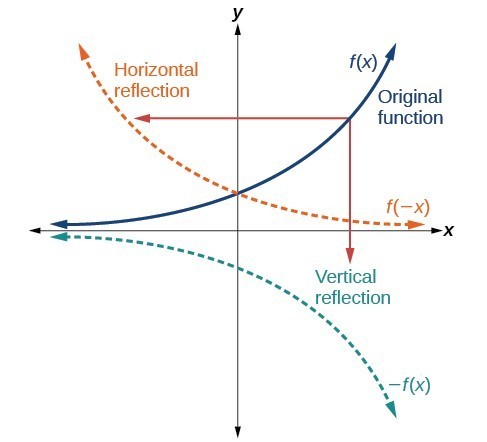
Another transformation that can be applied to a function is a reflection over the latexx/latex– or latexy/latexaxis A vertical reflection reflects a graph vertically across the latexx/latexaxis, while a horizontal reflection reflects a graph horizontally across the latexy/latexaxis The reflections are shown in Figure 9 Write a rule in function notation to describe the transformation that is a reflection across the yaxis A Rx0 (X,Y) B Ry0 (X,Y) C Ryx (X,Y) D Rx–1 (X,Y) Triangle ABC below is reflected across the yaxis and then translated 1 unit right and 2 units down A)Write the coordinated of the vertices of the image after reflection 1) y = f(x) (This is the reflection about the xaxis of the graph y = f(x))That is for every point (x, y) there is a point (x, y)Look at the example graphs below of y = x 2 and y = x 2Notice the green graph is simply the same as the blue graph folded down across the xaxis

Reflection Over The X And Y Axis The Complete Guide Mashup Math

Reflect Function About Y Axis F X Expii

Answer (1 of 3) Hey Fam One of the most basic transformations you can make with simple functions is to reflect it across the yaxis or another vertical axis In a potential test question, this can be phrased in many different ways, so make sure you recognize the following terms as just anotherAnother effect of "a" is to reflect the graph across the xaxis When the parent function f(x) = x 2 has an avalue that is less than 0, the graph reflects across the xaxis before it is transformed The graph below represents the function f(x) = x 2 In function notation, this reflection is represented by a negative outside the function f(x) If the negative is inside the function notationThis activity has 12 questions about reflections across the x and y axis The students are given a graph They read they ordered pair off the graph and reflect it across the x or y axis The 12 problems are multiple choice problemsThis is a digital copy to use with Google Subjects Math, Geometry Grades 5 th 9 th Types Assessment, Homework Also included in Transformations

Biomath Transformation Of Graphs

1

Using coordinates to describe position after reflection; Reflection in the coordinate plane is based on whether the reflection is over \(X\)axis, \(Y\)axis and in the origin \(\left( {0,\,0} \right)\) Reflection over Xaxis The \(x\)coordinate remains the same when a point is reflected across the \(x\)axis, while the \(y\)coordinate is turned into the opposite (its sign is changed) When graphing, if you forget theReflecting Functions Vertically or Horizontally Two types of transformations act like reflections or flips One transformation changes all positive outputs to negative and all negative outputs to positive The other reverses the inputs — positive to negative and negative to positive Reflecting downward puts all the points below the x axis

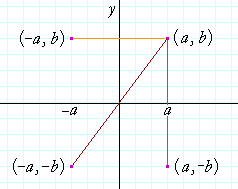
Reflections Of A Graph Topics In Precalculus

Reflect Function About X Axis F X Expii

Background Tutorials Translations What is an Image?A reflection over the xaxis can be seen in the picture below in which point A is reflected to its image A' The general rule for a reflection over the xaxis $ (A,B) \rightarrow (A, B) $ Diagram 3 Applet 1 You can drag the point anywhere you want Reflection over the yaxisReflecting shapes across the x axis and the y axis;

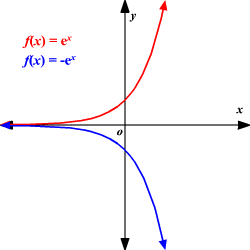
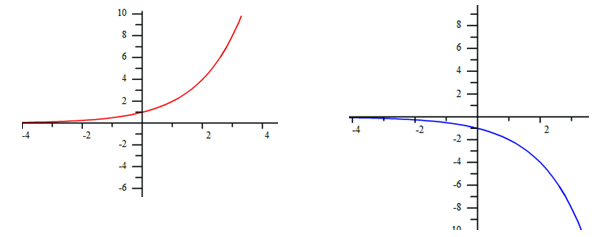
Graphs Of Exponential Functions

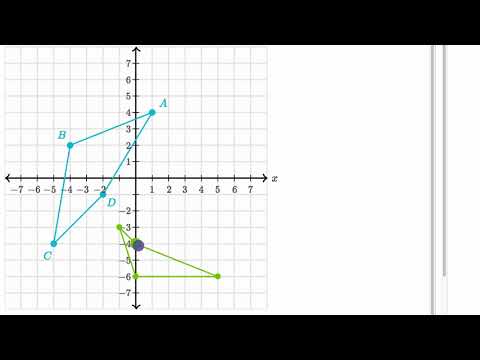
Reflecting Shapes Video Reflections Khan Academy

 Reflection over the xaxis is the process of producing a coordinate point that is mirrored across the xaxis of the coordinate plane That is it has the same X coordinate and opposite y4 coordinate Reflect over XAxis Example How to reflect over the xaxis?Identifying, describing & representing the position of a shape following a reflection;The xaxis is the horizon, and the tree is being reflected over the horizon, appearing upside down in its reflection In Game Maker, Flip X does not refer to flipping something along the xaxis, but instead to flipping the xcoordinates themselves While reflecting over the xaxis would negate the ycoordinates, Flip X negates the xcoordinates, making it in math terms a yaxis reflection (or

Assignment 2 Transforming Parabolas

How To Reflect A Shape In The X Axis Or Y Axis On A Coordinate Grid Youtube

Exploring missing lines of symmetry following a reflection;Another transformation that can be applied to a function is a reflection over the x– or yaxis A vertical reflection reflects a graph vertically across the xaxis, while a horizontal reflection reflects a graph horizontally across the yaxis The reflections are shown in Figure 9 Figure 9 Vertical and horizontal reflections of a function Notice that the vertical reflection produces aAs you can see, the graph of y 2 (x) is in fact the base graph g(x) reflected across the xaxis Reflections Across the yAxis You can visualize a reflection across the yaxis by imagining the graph that would result from folding the base graph along the yaxis Symbolically, we define reflections across the yaxis as follows Definition For the base function f (x) , the function

How To Reflect A Graph Through The X Axis Studypug

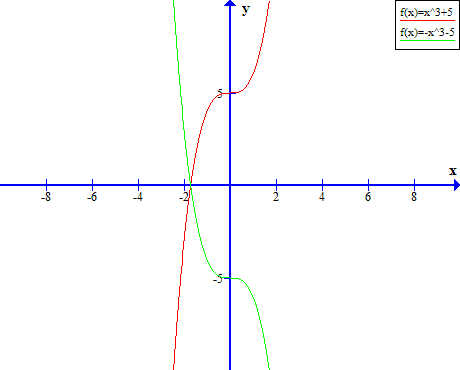
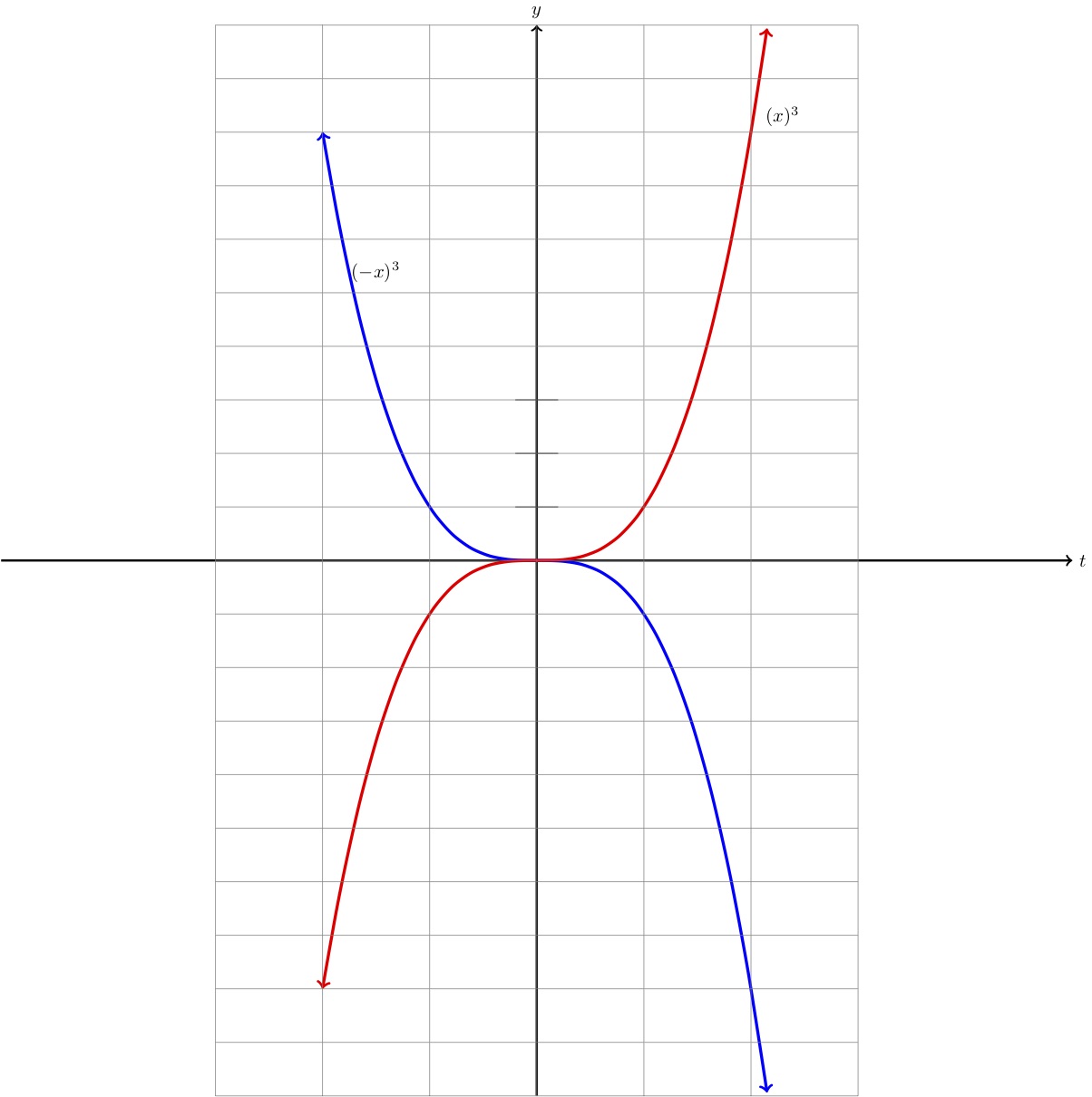
If The Graph Y X 3 5 Is Reflected In The X Axis What Is The New Equation Socratic

Functions of graphs can be transformed to show shifts and reflections Graphic designers and 3D modellers use transformations of graphs to design objects and imagesRewriting the equation to reflect it across either the 'x' or 'yaxis' If g(x) is a function, then (1)*g(x) →g(x) →reflection across 'xaxis' If k(x) is a function, then k(1*x) →k(x) →reflection across 'yaxis' Math1050 Session 7 (Textbook 35) Graphical Transformations Transformation an adjustment made to any function that results in a change to the graphThis video explains to graph graph reflections across the xaxis and yaxis in the form a*f(b(xc))d This video looks at how the sign of a and b affect th

Transformations Of Functions Mathbitsnotebook A1 Ccss Math

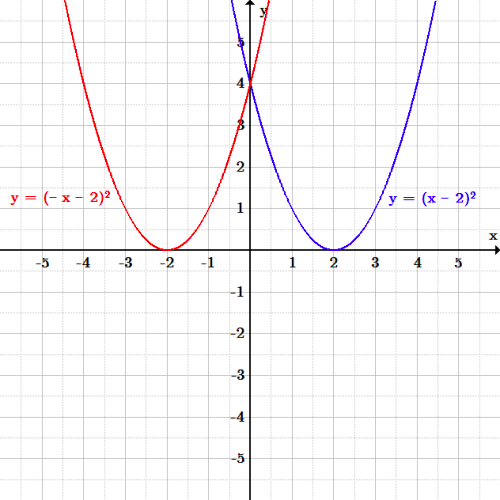
Transformations Boundless Algebra

The reflection transformation may be in reference to X and Yaxis Reflection over Xaxis When a point is reflected across the Xaxis, the xcoordinates remain the same But the Ycoordinates are transformed into its opposite signs Therefore, the reflection of the point (x, y) across Xaxis is (x, y) Reflection over Yaxis When a point is reflected across the Yaxis, the YReflecting a figure over the yaxis can be a little tricky, unless you have a plan In this tutorial, see how to use the graph of a figure to perform the reflection Check it out!Determine the coordinate points of the image after a reflection over the xaxis Since the reflection applied is going to be over the xaxis, that means negating the yvalue As a result, points of the image are going to be A'(1,2), B'(3,5), and C'(7,1) By counting the units, we know that point A is located two units above the xaxis Count two units below the xaxis and there is

How To Reflect A Graph Through The X Axis Studypug

Reflecting Functions Or Graphs Examples Solutions Worksheets Videos Games Activities

Practice Reflecting a Line Segment Across the Xaxis or Yaxis with practice problems and explanations Get instant feedback, extra help and stepbyXaxis reflection A reflection across the xaxis changes the position of the ycoordinate of all the points in a figure such that (x, y) becomes (x , y) Triangle ABC has vertices A (6, 2), B (4, 6), and C (2, 4) Triangle DEF is formed by reflecting ABC across the xaxis and has vertices D (6, 2), E (4, 6) and F (2, 4) All of the points on triangle ABC undergo the same change toExploring reflections and translations (Part 1) Exploring reflections and translations

Mfg Reflections And Even And Odd Functions

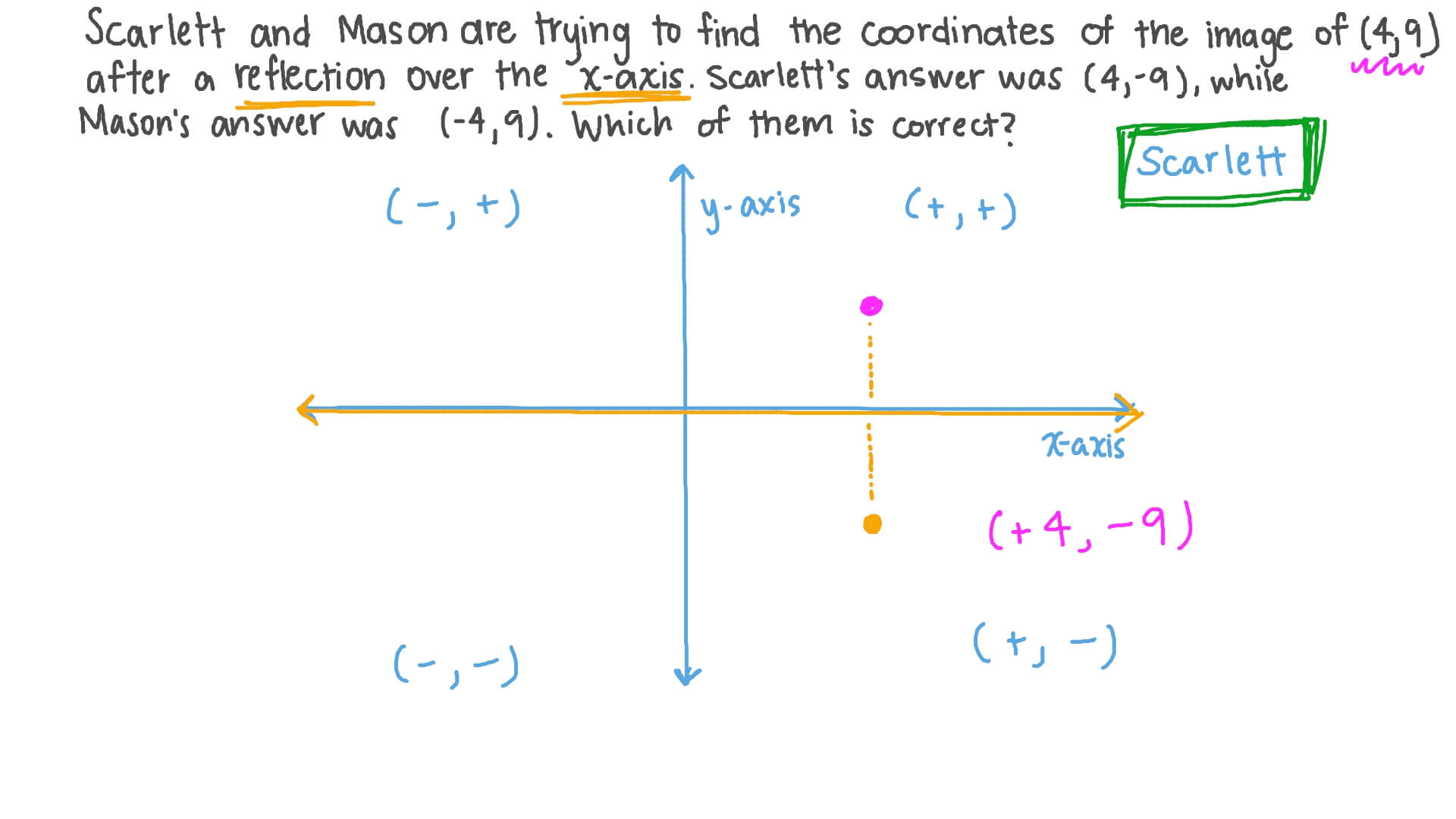
Question Video Determining The Coordinates Of A Point After Its Reflection Over The 𝑥 Axis Nagwa

Reflecting a figure over the xaxis can be a little tricky, unless you have a plan In this tutorial, see how to use the graph of a figure to perform the reflection Check it out! Answer You could plot the point and work out the answer quite rapidly I'll do this and the matrix method If we reflect (4,3) across y axis, the point becomes (4,3) if you visualise it When we reflect this in the 'x' axis, we get (4,3), if you visualise it This is a lot faster than the(Reflection across the xaxis) Whenever the minus sign () is in front of the function notation, it indicates a reflection across the xaxis For example, the graph of f(x) is a reflection of the graph of f(x) across the xaxis The graph of 3 g(x) involves the reflection of the graph of g(x) across the xaxis and the upward shift of the reflected graph 3 units Example 1 Graph the

Reflection Across The Y Axis Math Functions Showme

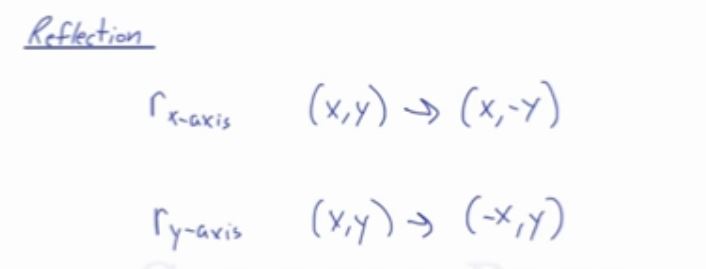
Learn About Reflection Over An Axis Over X Axis Or Y Axis

 Reflection over the xaxis for Sets of Coordinates (x, y), Functions, Coordinates (with Matrices) 1 Reflection Over The XAxis Sets of Coordinates If you have a set of coordinates, place a negative sign in front of the value of each yvalue, but leave the yvalue the same Example question #1 Reflect the following set of coordinates over the xaxis (4, 6), (2, What are the coordinates of the reflection of the point 3/4 across Y axis?REFLECTION OVER X AXIS AND Y AXIS When P(x, y) is reflected in the mirror line to become p'(x', y'), the mirror line perpendicularly bisects pp' Thus, for every point of an object, the mirror line is perpendicularly bisects the line segment joining the point with its image Reflection on x axis Reflection on y axis Reflection about y = x Reflection about y = x Example 1 Find the

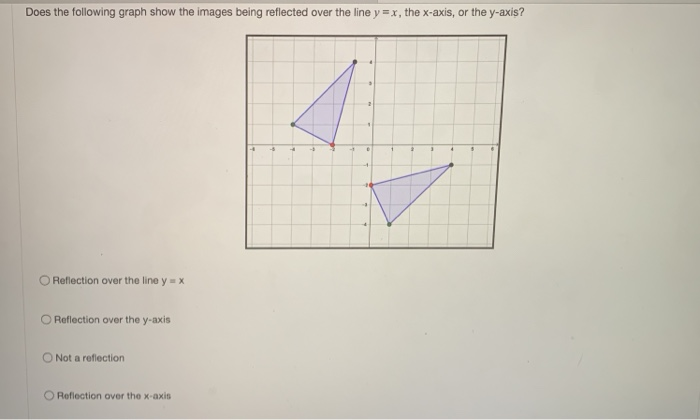
Solved Does The Following Graph Show The Images Being Chegg Com

Reflections Of Functions Justin Skycak

Reflection across the yaxis changes the sign of the x coordinate only, How to reflect shapes on a mirror line or x axis, y axis, coordinate grid, graph or even cartesian plane – it's all pretty much the same The biggest problem isn't really the reflection it's more making sure that you've correctly identified the 'mirror line' This can cause a few problems and a little confusion The easiest way is to actually plot a couple of points on theReview how to reflect objects across the x and y axis on the coordinate plane by following simple rulesThis lesson is given by Taina MaisonetYou can follow

Does Reflecting The Parabola Across The X Axis Change The Axis Of Symmetry Quora

Biomath Transformation Of Graphs

Did you know that//example slope from line y = mx 0 var tmpMatrixName Email address You answer Send answer Related questions and answers What is a reflection point on a graph?

Stretching And Reflecting Transformations Read Algebra Ck 12 Foundation

Reflection Mathbitsnotebook A1 Ccss Math

If we look the location of the point on the graph, it is located at x=3, y=4 To find the reflection, we need to cross the y axis which would be x=3 The y coordinate will be the same since we are crossing the x axis and the point will still be above the xaxis For a reflection over the x axis, leave the x coordinate unchanged and change the sign of the y coordinateFor a reflection over the y axis, leave the y coordinate unchanged and change the sign of the x coordinate How does a reflection across the y axis change the coordinates of a point?Then we want to reflect about the X axis Robert, when you reflect about the X axis, you want to exclude the minus sign or the negative science So we exclude the negative sign and it reflects across the X axis And then if we want to reflect across the Y axis, we put it on the inside that are about to cross the y axis Then if we want to

Transformations Reflection Across The X Axis Youtube

Why Isn T F X Sqrt 2 X Reflected Across The Y Axis Mathematics Stack Exchange

Functions of graphs can be transformed to show shifts and reflections Graphic designers and 3D modellers use transformations of graphs to design objects and images Reflection Over the XAxis For our first example let's stick to the very simple parent graph of y = x^2 {See video for graph} On the screen you can see that the graph ofThe best way to practice drawing reflections over y axis is to do an example problem Example Given the graph of y = f (x) y=f(x) y = f (x) as shown, sketch y = f − x) y = f(x) y = f (− x) Remember, the only step we have to do before plotting the f(x) reflection is simply divide the xcoordinates of easytodetermine points on our graph above by (1) When we say "easyto

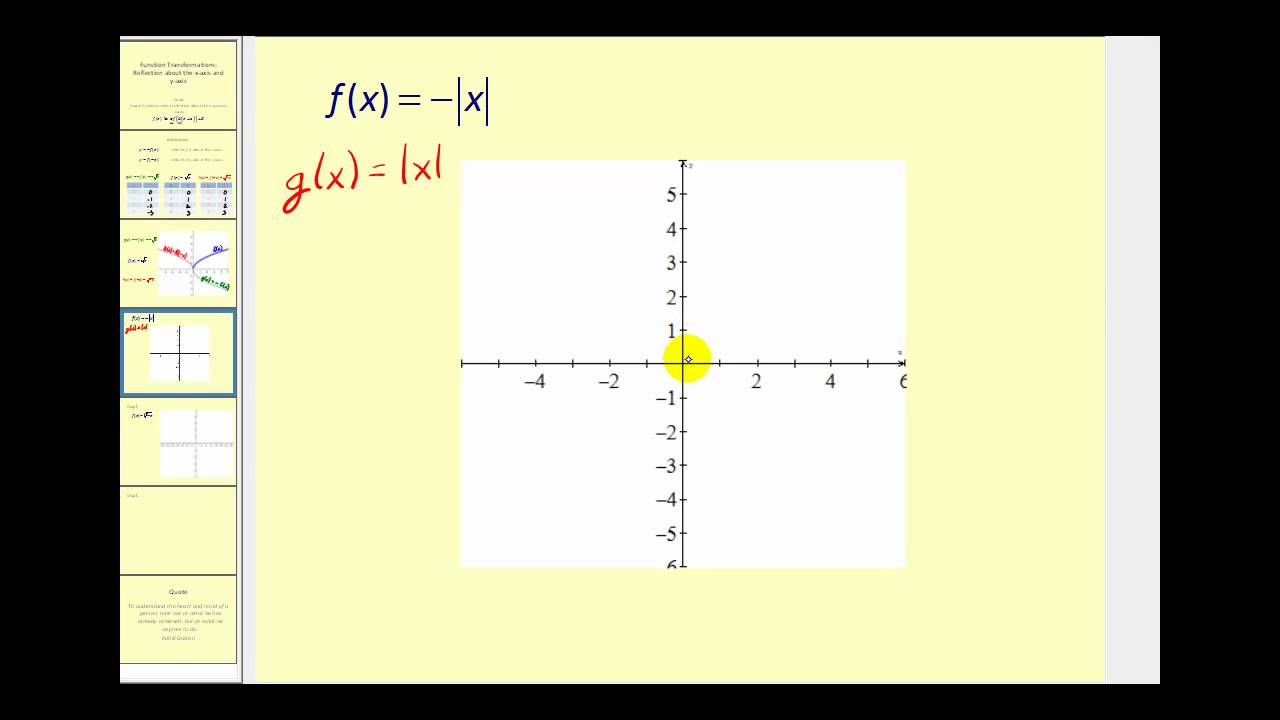
Function Transformations Reflections Across The X Axis And Y Axis Youtube

Graphing A Square Root Function With A Horizontal Vertical Shift And Reflection Over X Axis Youtube

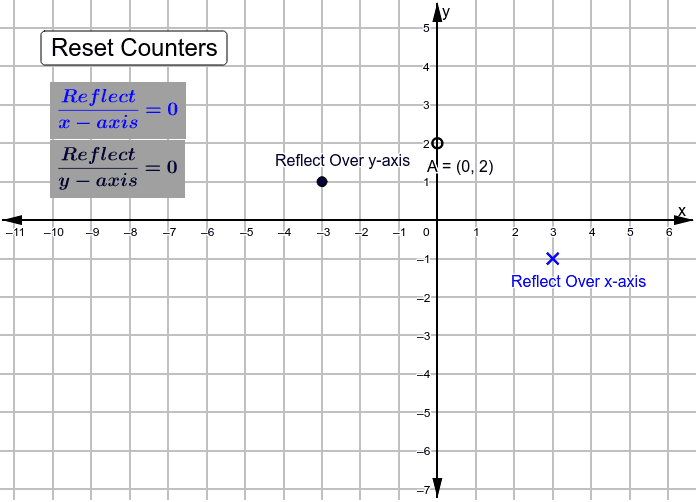
Reflect Points Across X Axis Y Axis Geogebra

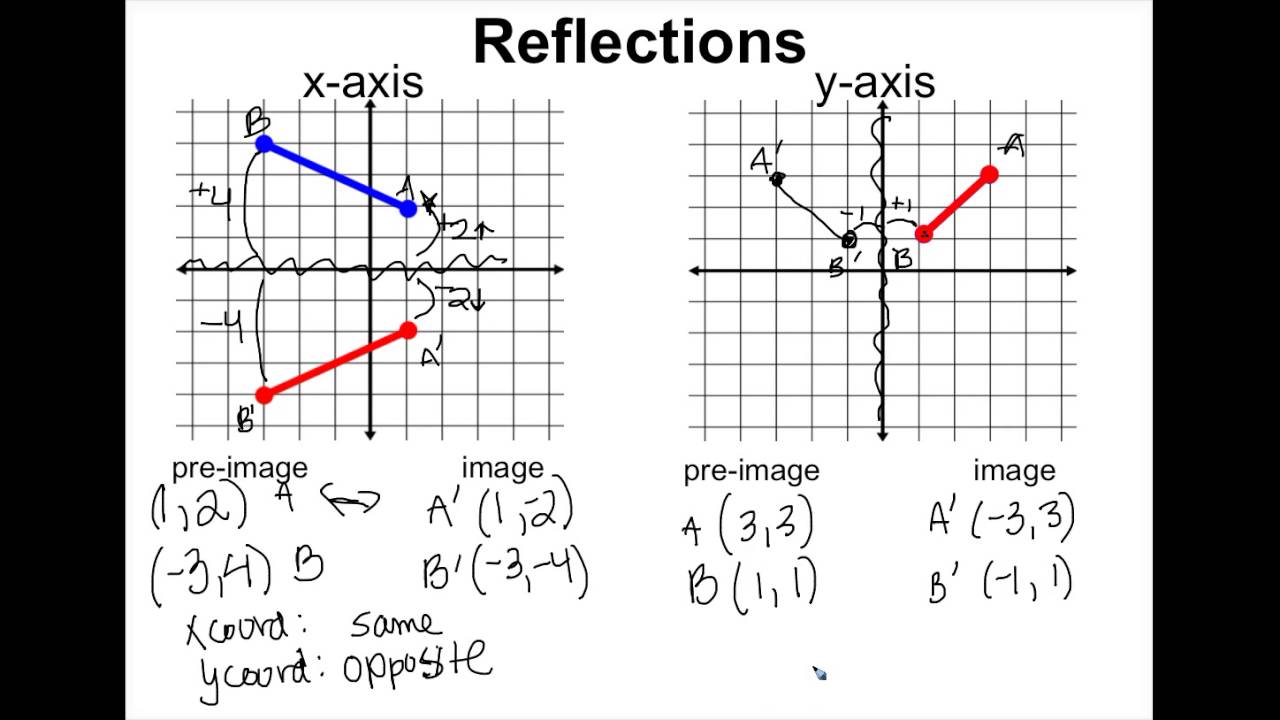
Transformations Reflections X And Y Axis Youtube

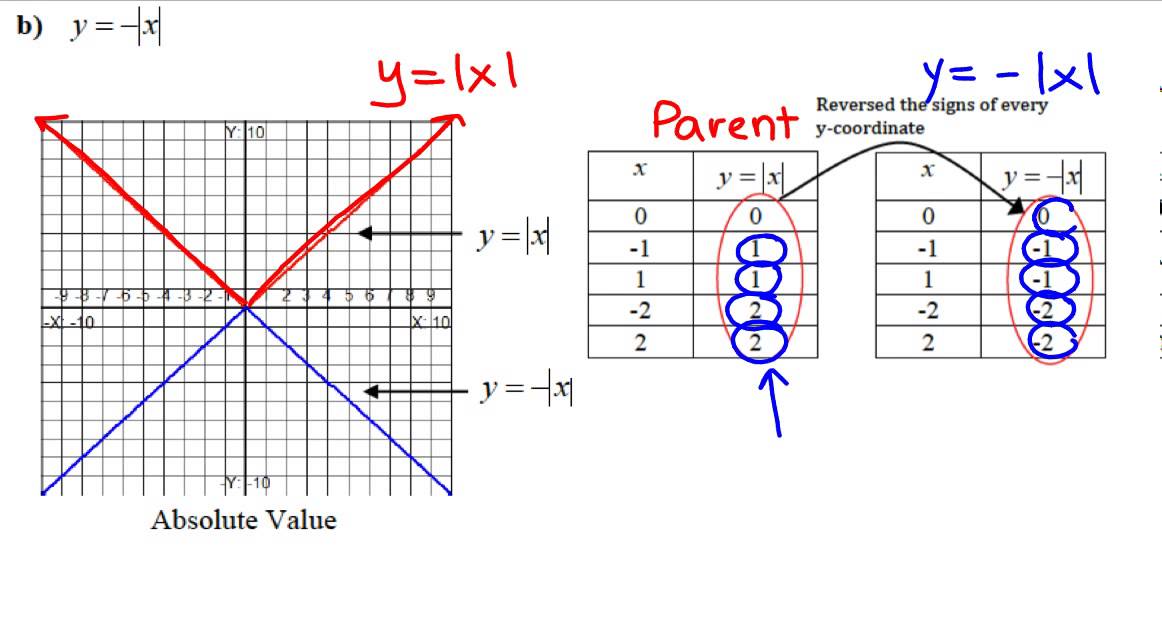
Transformations Of Reflections About The X And Y Axis Youtube

Untitled Document

Transformations Reflecting Over The X Axis

Assignment 2 Transforming Parabolas

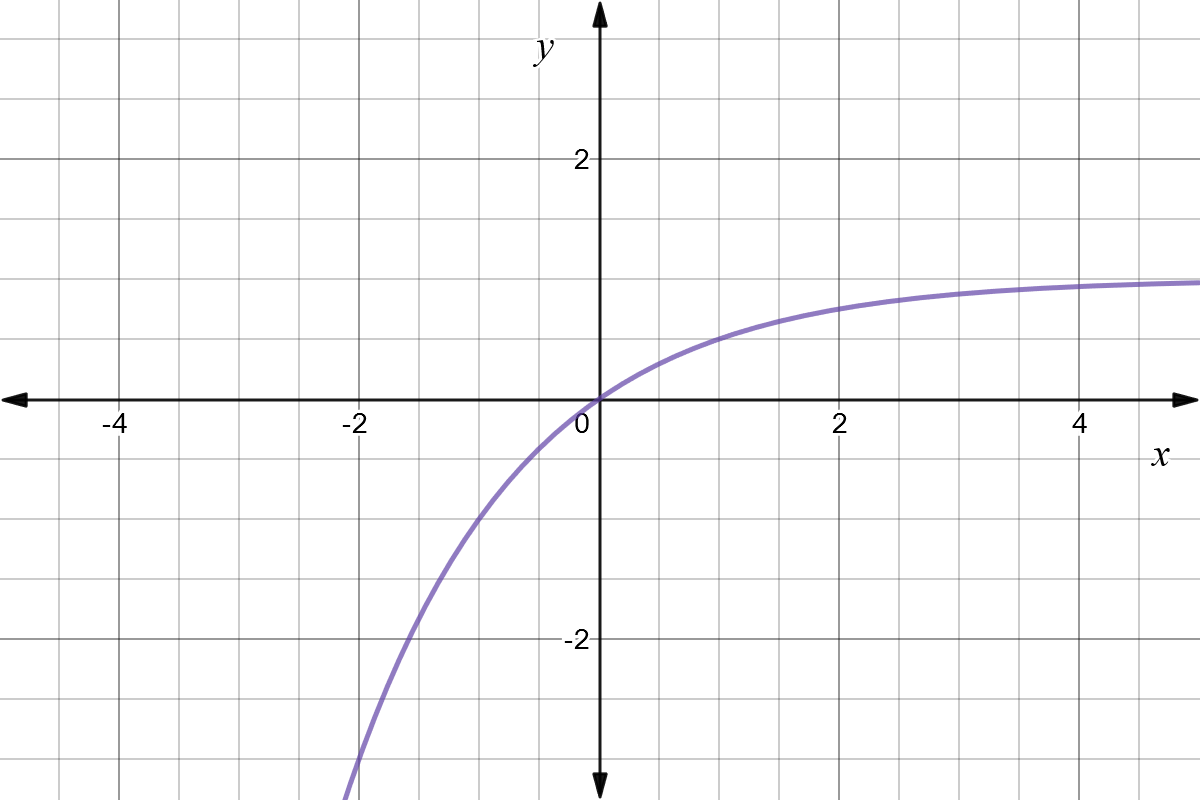
A Review Of Logarithms

How To Reflect A Graph Through The X Axis Y Axis Or Origin Interactive Mathematics

How To Reflect Quadratic Equations Video Lesson Transcript Study Com

Reflections Across The X And Y Axis Geogebra

Reflect Function About Y Axis F X Expii

Reflection Across The X Axis Reflection Math Chart

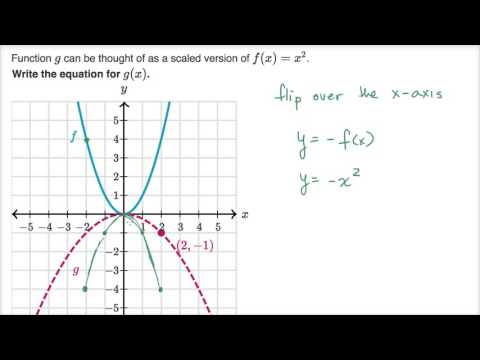
Scaling Reflecting Parabolas Video Khan Academy

Solved Is F X A Reflection Over The X Or Y Axis Explain

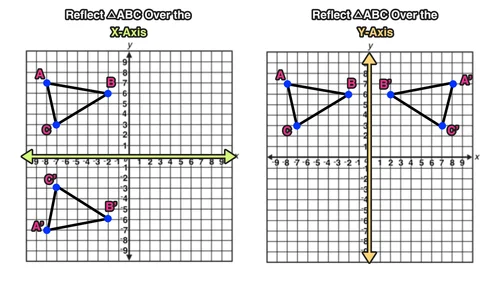
Reflection Over The X And Y Axis The Complete Guide Mashup Math

Reflection Rules How To W 25 Step By Step Examples

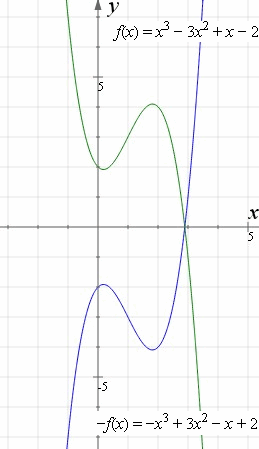
How To Reflect A Graph Through The X Axis Y Axis Or Origin Interactive Mathematics

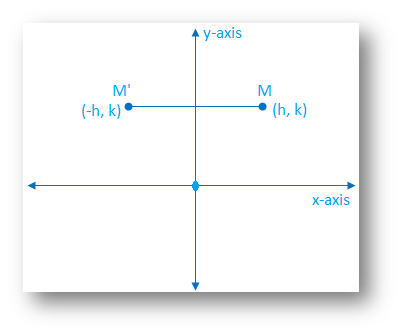
Reflection Of A Point In Y Axis Reflection Of A Point Reflection

Graph Functions Using Reflections About The X Axis And The Y Axis College Algebra

How To Find A Reflection Image

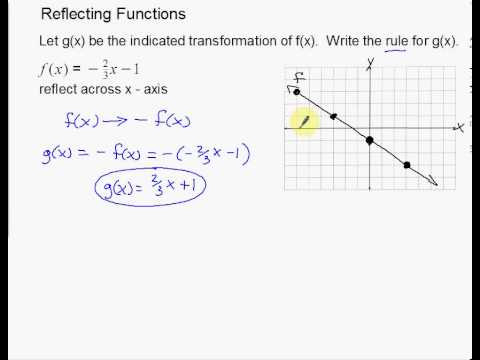
Linear Reflections Across X And Y Axis Example Youtube

Transformations Boundless Algebra

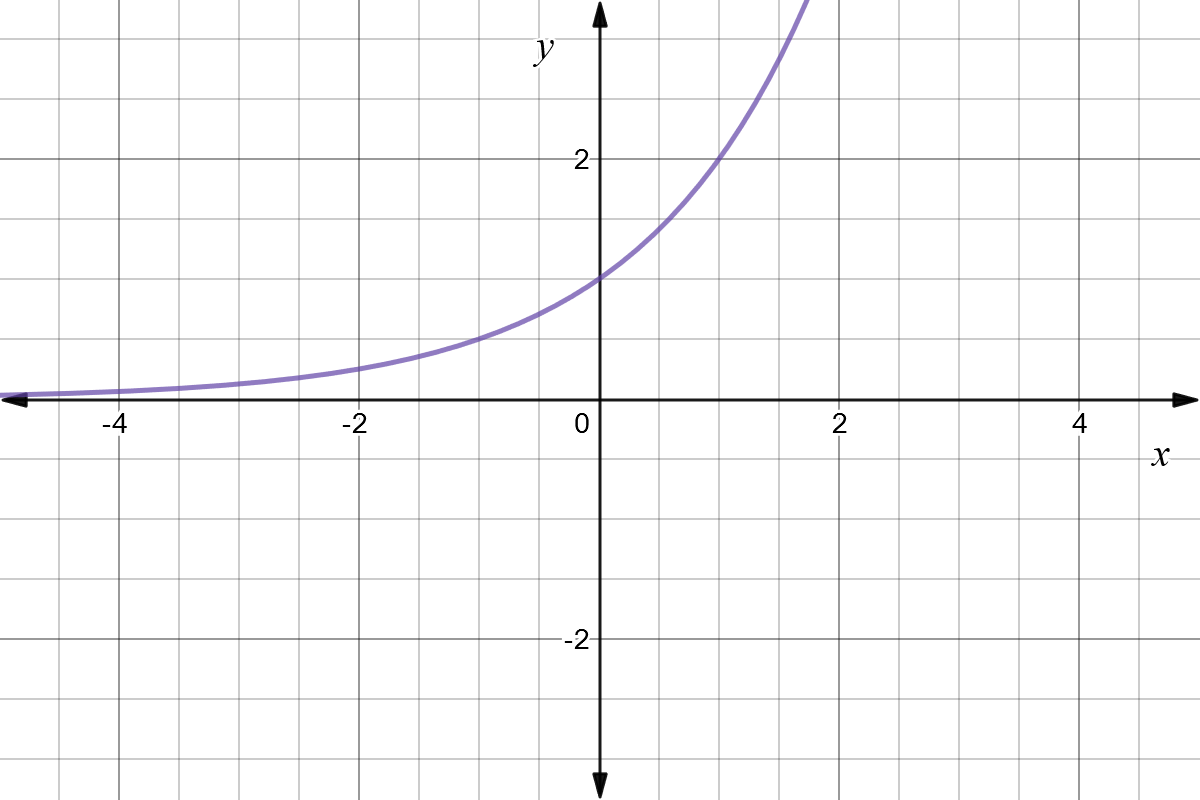
Reflect Function About Y Axis F X Expii

1

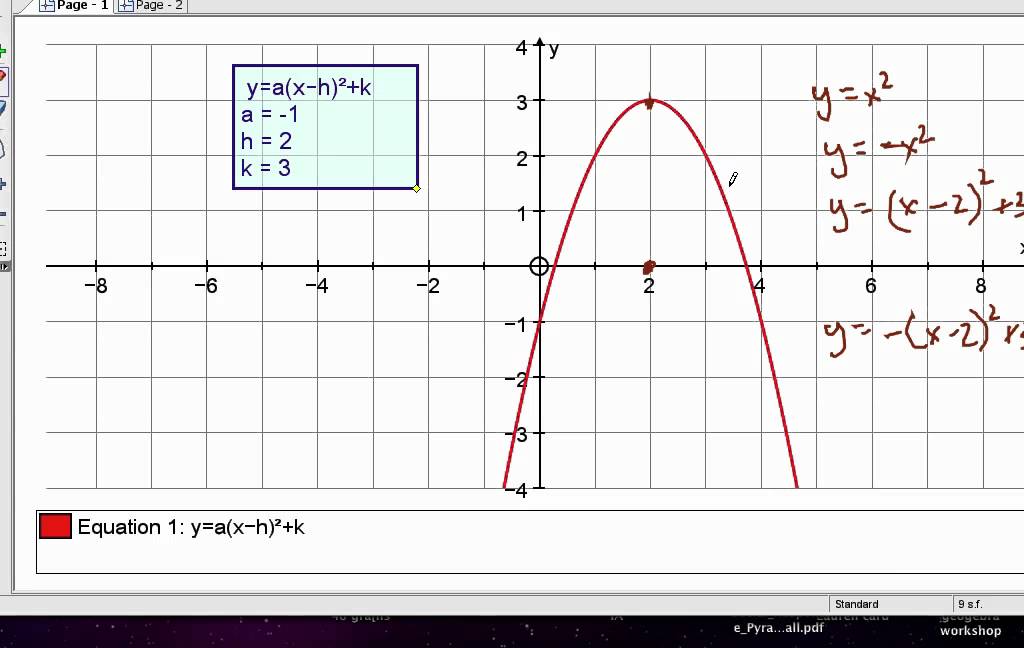
2 02 0 Reflection Over X Axis Mov Youtube

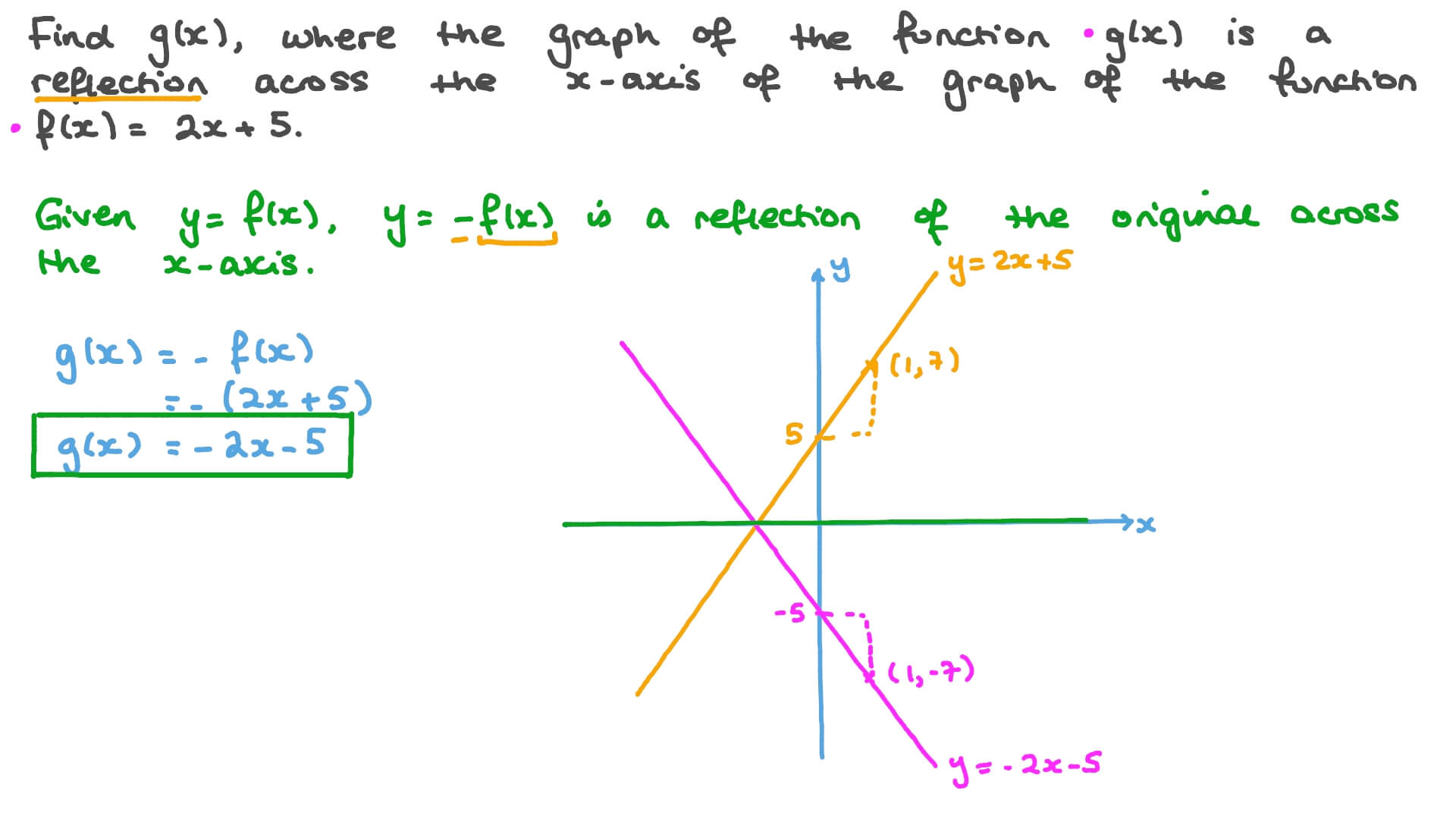
Question Video Identifying The Equation Of A Graph After A Reflection Nagwa

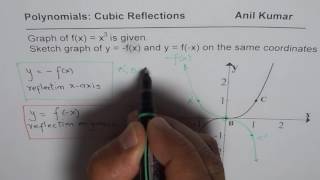
Reflection Of Cubic Function On X And Y Axis Results In Same Graph Youtube

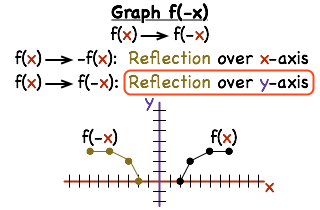
How Do You Graph A Reflection Of A Function Printable Summary Virtual Nerd

Transformation Of Function

Determine Whether A Function Is Even Odd Or Neither From Its Graph College Algebra

Reflection Through X Axis

Reflect Points Over The X And Y Axes Learnzillion

Reflections Math Tastic Concepts For 7th Grade

How To Reflect A Line Segment Across The X Axis Or Y Axis Geometry Study Com

Transformations Reflecting Across The X Axis

Describe The Transformation A Reflection Across The X Axis B Reflection Across The Y Axis C Brainly Com

Solution The Line With The Equation Y 2x 3 Is Reflected In The Y Axis Find The Equation Of The Image Line

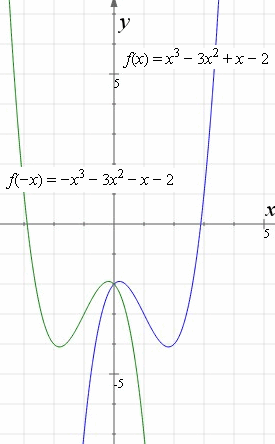
How To Reflect A Graph Through The X Axis Y Axis Or Origin Interactive Mathematics

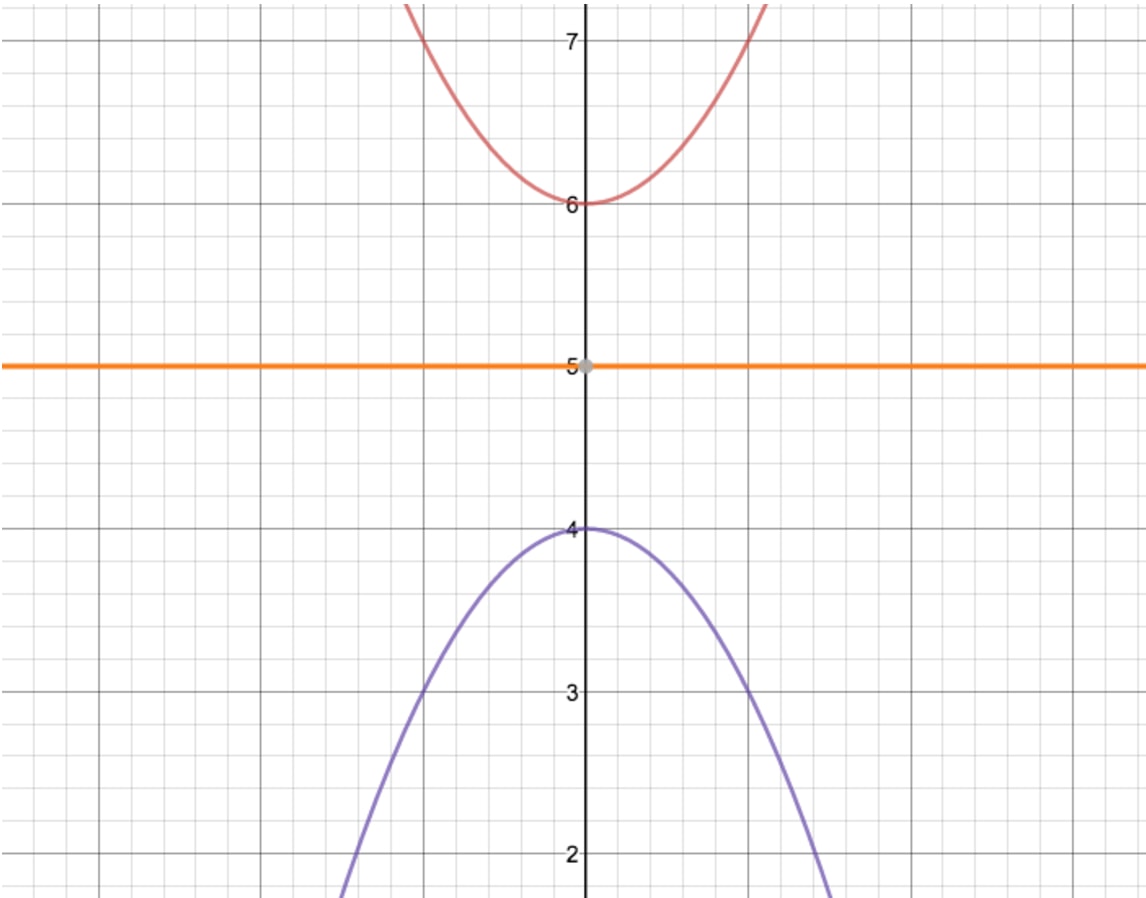
How To Reflect A Graph Through The X Axis Studypug

Reflection

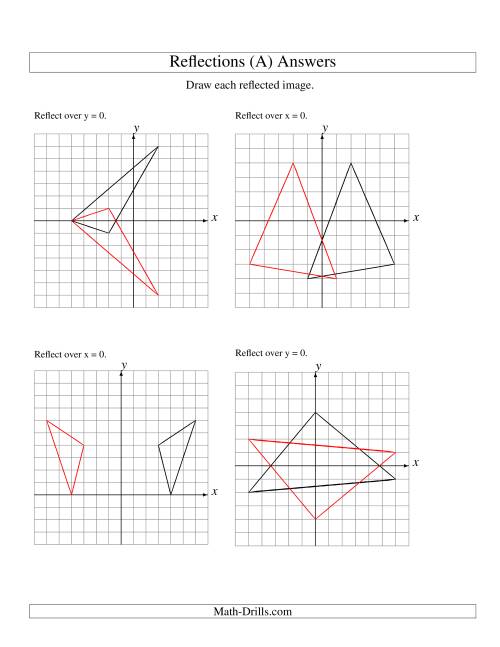
Reflection Of 3 Vertices Over The X Or Y Axis A

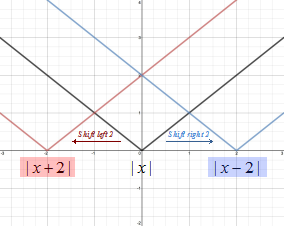
Function Transformations

1

Why Aren T Reflected Lines Perpendicular Meaning Why Are Their Slopes Negative Not Negative Reciprocals Enotes Com

Reflect Triangle Over X Axis Y Axis Y X Y X Geogebra

Stretching And Reflecting Transformations Read Algebra Ck 12 Foundation

How To Graph Reflections Across Axes The Origin And Line Y X Video Lesson Transcript Study Com

Transformation Reflection Over X Axis

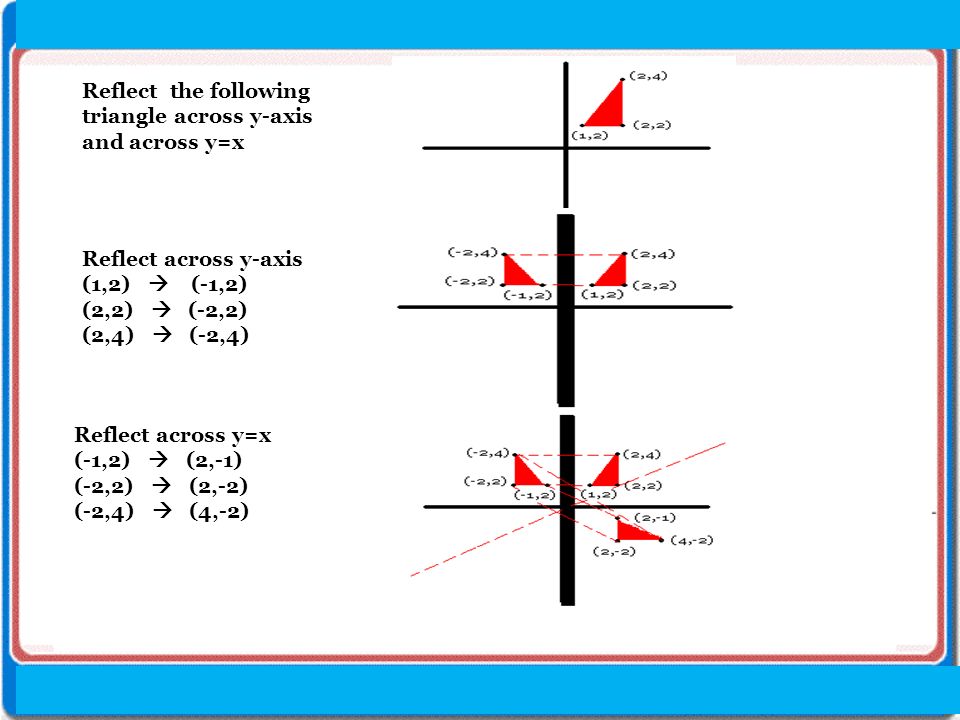
Reflections 30 Reflect Across Y X X Y Y X Reflect Across X Axis X Y X Y Reflect Across Y Axis X Y X Y Reflect Across Y X Reflect Across Ppt Download

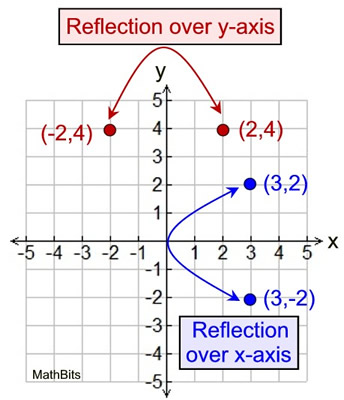
Graphing Coordinate Axes Mathbitsnotebook Jr

Assignment 2 Transforming Parabolas

Reflections In Math Formula Examples Practice And Interactive Applet On Common Types Of Reflections Like X Axis Y Axis And Lines

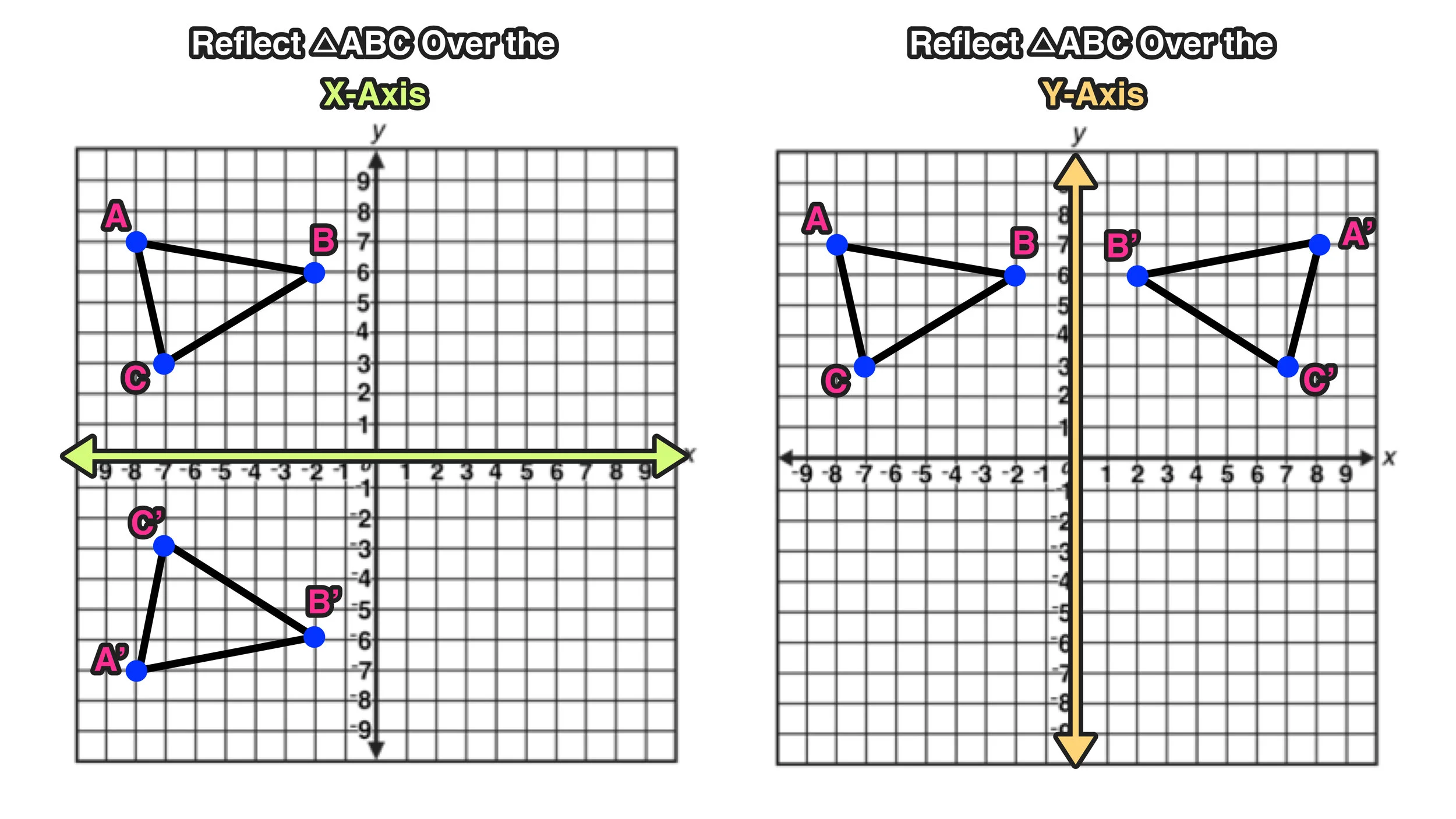
Reflection Over The X And Y Axis The Complete Guide Mashup Math

A Rotation Of 180º And Then A Reflection Across The X Axis B Reflection Over The X Axis And Then Brainly Com

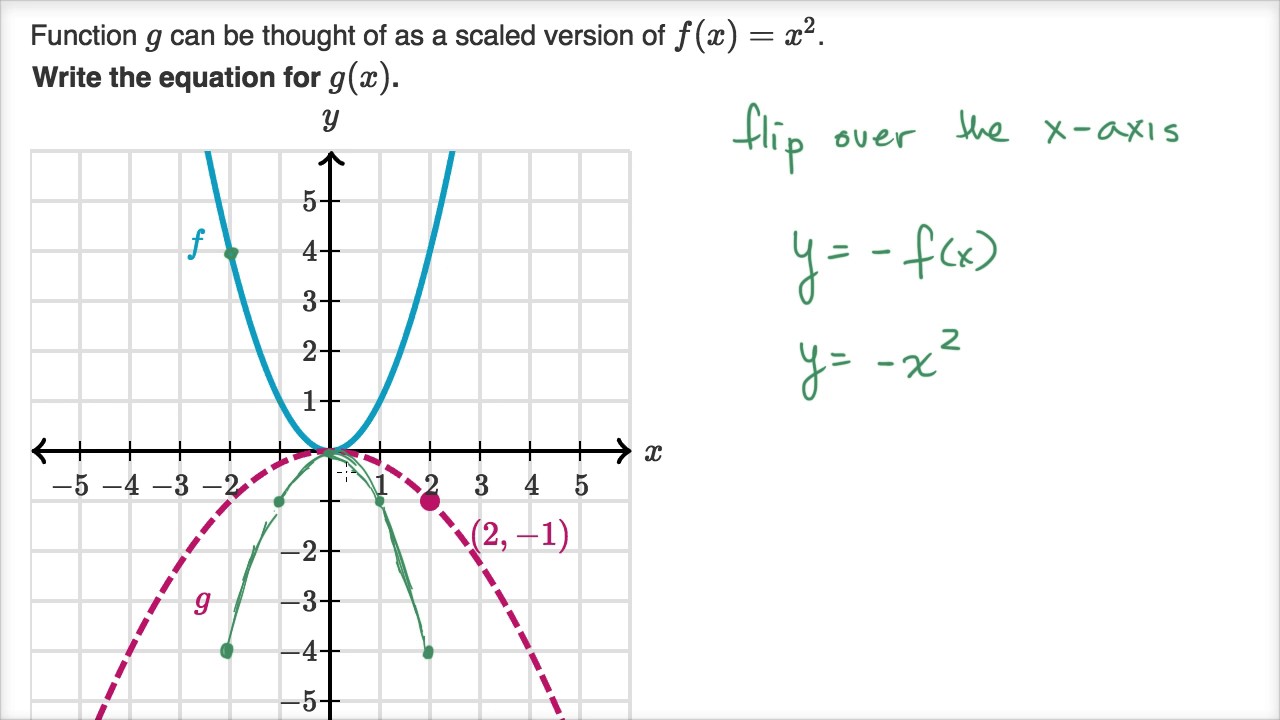
Scaling Reflecting Parabolas Video Khan Academy

A Review Of Logarithms

Reflections Math Analysis Functions

How To Reflect Quadratic Equations Video Lesson Transcript Study Com

Biomath Transformation Of Graphs

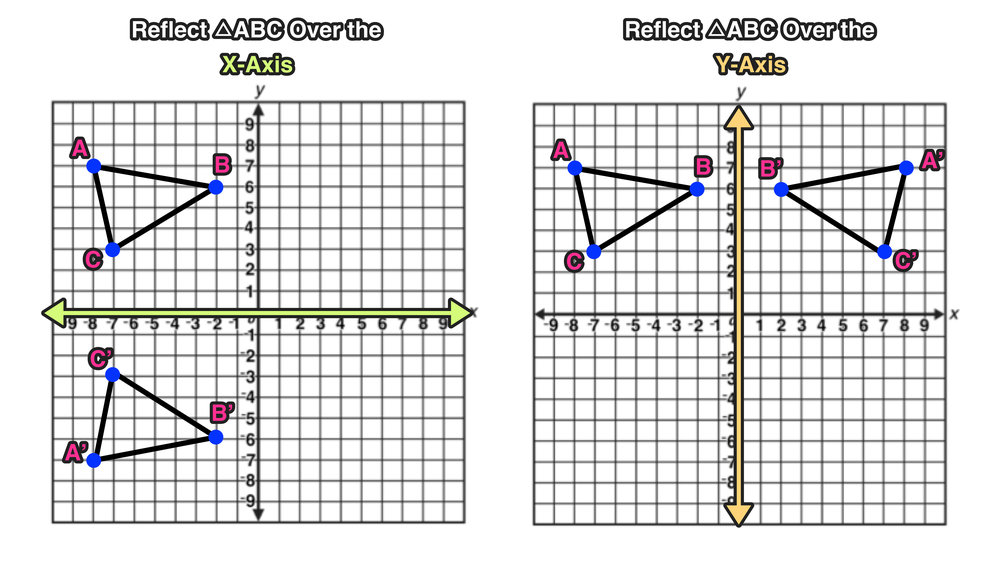
Reflection Over The X And Y Axis The Complete Guide Mashup Math

Reflections

How To Reflect A Graph Through The X Axis Y Axis Or Origin Interactive Mathematics

Transformations Of Functions Mathbitsnotebook A1 Ccss Math

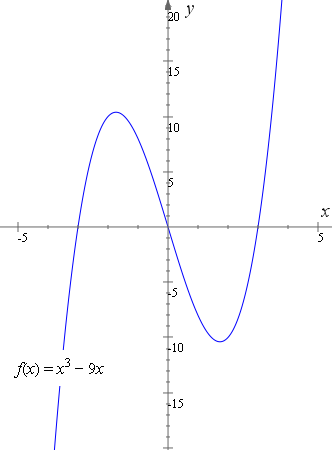
How To Reflect A Graph Through The X Axis Y Axis Or Origin Interactive Mathematics

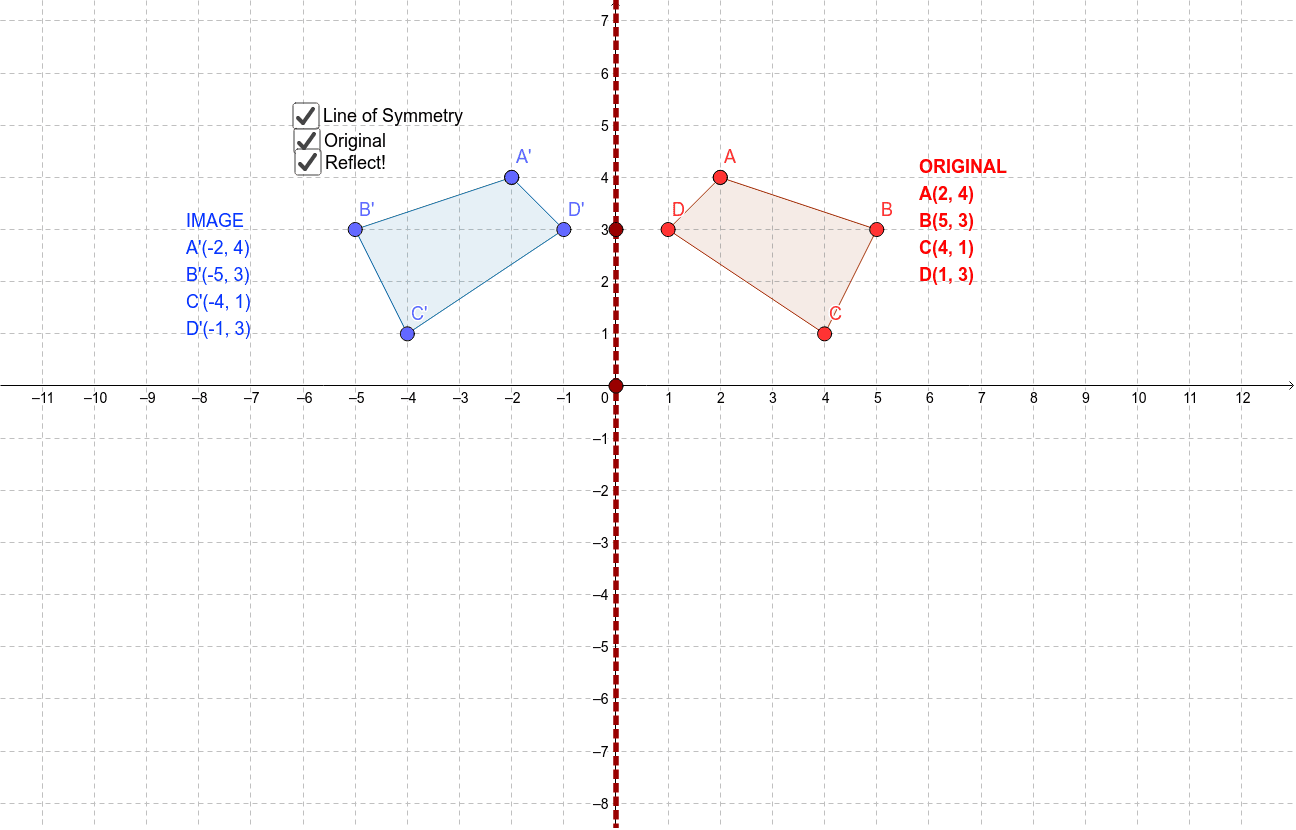
Transformation Reflection Geogebra

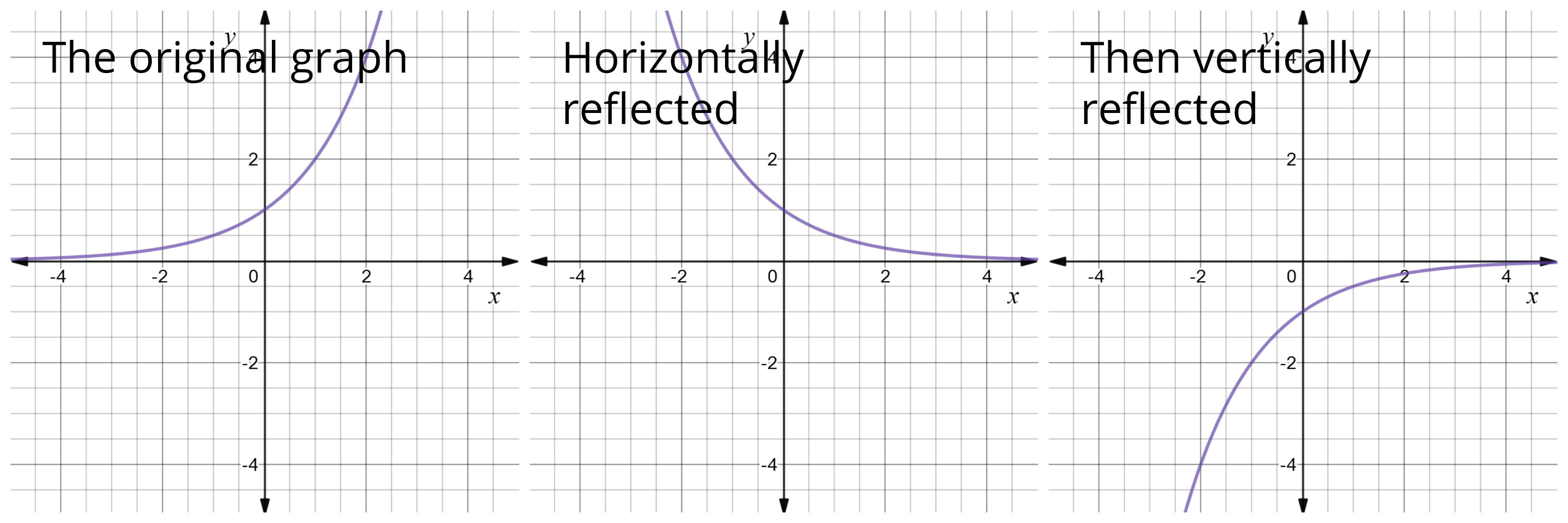
Reflect Function About Y Axis F X Expii

Reflections Through The Axes And The Lines Y X And Y X Geogebra

Stretching And Reflecting Transformations Read Algebra Ck 12 Foundation

Graph Functions Using Reflections About The X Axis And The Y Axis College Algebra

Reflections Of A Graph Topics In Precalculus

What Is The Reflection Over The Y Axis Of The Point 4 7 Quora

1

Incoming Term: reflection across x axis vs y axis, reflection across x axis and y axis, reflection across the y axis vs x axis,